Student Problem #2

This video shows my boy Jonathan and myself solving a logarithmic problem that we created. This type of problem is found in our Unit H SSS packet and more specifically in the "finding logs given approximations" portion which is Concept 7.

Unit G Summary Question #10: Range

While the domain of a rational function depends on DIVAH, what do you think the range of a rational function depends on? Give an example.

Because a hole is a point with a x and a y value, a hole would be an area where the range of a rational function is limited. A horizontal asymptote is an imaginary horizontal line so that means all the values on a horizontal asymptote is another limit to the range of a rational function.

Unit G Summary Question #9: X-intercept

Describe how to find the x-intercepts of a rational function. Include both the long way and the shortcut way, explaining why the shortcut makes mathematical sense.

To find the x-intercept, you have to plug in 0 for y in the factored equation, both the numerator and the denominator. That is the "long" way. The shortcut way would be just plugging in 0 for the numerator. This makes sense because if you plug in 0 for the denominator, the denominator will eventually cancel after it is multiplied to zero which is done to get the numerator by itself.

Unit G Summary Question #8: Y-intercept

How do you find the y-intercept of a rational function? Does this need to be done in the original or simplified equation?

To find the y-intercept of a rational function, simply plug in 0 for all the x's in the function. It should be done with the simplified equation but it is possible to do it with the original one.

Unit G Summary Question #7: Limit Notation for VAs

Describe how to write limit notation for vertical asymptotes and what the notation means.

To write limit notation for vertical asymptotes, a graphing calculator is required. The first part of the limit notation (as x-->_) has to do with what the equation/equations is/are. If the equation is x=2, then the x--> part will have 2. The second part needs a graphing calculator because it will tell you the function will be at the right or the left of the vertical asymptote and whether it will go up or down.

Unit G Summary Question #6: Holes

How do we find the appropriate place to plot a hole if the y-value is undefined when plugged into the original equation?

First one must need to know how to find a hole in order before I can go any further. You find the hole, which is a point, by plugging in the number you get for a vertical asymptote into the factored equation that has not been cancelled. You will get a value and that value will be the y-value of your hole. Now sometimes the y-value is undefined when a hole is plugged into the original equation. This will not matter because you are supposed to plug in the hole into the simplified/not cancelled equation which will give you a rational/real y-value as your answer.

Unit G Summary Question #5: Asymptote Exceptions

Describe the conditions in which a graph can cross through an asymptote.

It is true that graphs are not supposed to cross an asymptote, there is an exception to this as there is with a lot of rules in this world. Graphs can cross through an asymptote, specifically a horizontal or a slant one. A graph can cross through a horizontal one towards the far right or far left of the graph. A graph can cross through a slant one towards the middle of the graph. They will only cross through a graph slightly, however. It is impossible for a graph to cross a vertical asymptote.

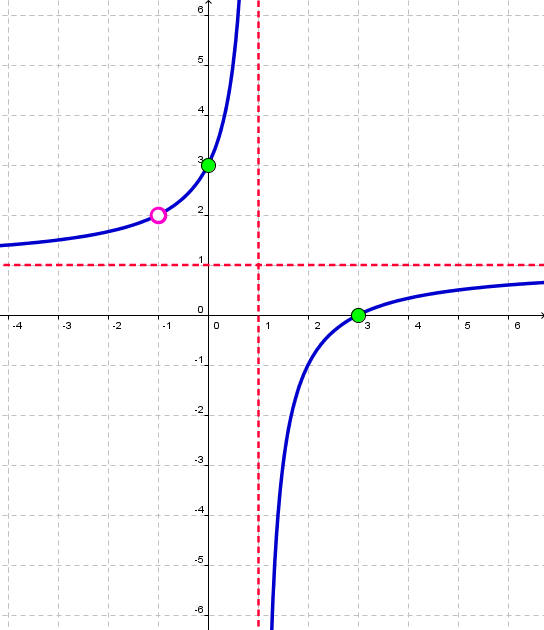
Unit G Summary Question #4: Vertical Asymptotes and Holes

What is the difference between a graph having a vertical asymptote and a graph having a hole?

A graph that has a vertical asymptote means that a function can approach but never touch that vertical asymptote. A graph that has a hole means that a function will get close to it, skip it, and continue on as if it were there but you actually do not touch it. The image on the left shows a vertical asymptote as a red dashed line.The image on the right shows a few holes in the functions. The every unusual function with a numerator and denominator will have a vertical asymptote whereas a hole only exists if something cancels when factoring the function.